|
The set of neutral diatonic modes
The author lays out the functions and main features of the neutral diatonic modes - a small set of useful symmetrical harmonies derived from the seven traditional diatonic modes.
For many years I have been exploring extended meantone harmony through my 31-et (31-tone equal tempered) guitar. Among the patterns that I have found pleasing sit a group of scales somewhat like the standard diatonic norms. I found a few of them ad hoc through the guitar, but then I discovered that I could systematically generate the whole set through a judicious examination of the circle of fifths. Given some preliminary conditions (the imposition of symmetry) it turned out that the set is really quite small: only three interval structures, each of which embodies the usual seven modes, making twenty-one in all. I devised a way of designating them that relates to their parentage. In this way they have become familiar to me. Leo de Vries, who is making his own extensive survey of symmetrical 31-et and 24-et harmonies, encouraged me to write this paper. I dedicate it to him.
These new modes are formed by bringing elements from the antipodal region into the diatonic strucure. Thus we can best begin by reviewing the familiar modes. These are:
L = 1 2 3 #4 5 6 7 I present these harmonies as modes rather than as scales because I am interested in function rather than pitch. I assume that the reader apply them to any tonality or pitch reference. The letter designation (in bold) comes from the familiar Greek names. L stands for the Lydian major mode. I indicates the Ionian major, often called the natural major mode. M designates the Mixolydian major. D gives the Dorian minor. A refers to the Aeolian minor, often called the natural minor mode. P stands for the Phrygian minor. Finally, O designates the less stable Locrian minor mode. Here I employ O since L has already been used. These letters act as convenient shorthand names for the rather cumbersome traditional names. They also enable further designation of the new modes. We must remember that these modes embody a single interval structure (the Diatonic Heptad). The function varies according to where we put the tonic. For example, take the white keys of the piano: FGABCDEF. This single set of pitches act as L if the tonic is on F, as I if the tonic is on C, and so on. Moreover, this interval structure is symmetrical. (Symmetry describes the ability to generate an identical interval sequence both up and down in direction). We can say, for example, that I has point symmetry (symmetry around a tone) centered on the function 2. More importantly, D has point symmetry around the tonic function 1. I will refer to this feature as tonic symmetry. The D mode sits in the middle of the entire group. The other modes arrange themselves around it in a spectrum between "more major" and "more minor". We will find similar features in the neutral diatonics.
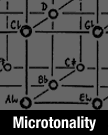
The foreign elements come from the antipodal region, so we must be clear about the meaning of the word "antipode". This term refers to an aspect of the circle of fifths - that place so far as possible removed from the tonic. For example, take the circle of fifths for 12-et. Here the antipode is the tritone (#4/b5). It sits maximally remote from the tonic in either direction around the circle. This interval has peculiar properties important for understanding the character and syntax of 12-et harmony. In the case of the circle of fifths for 31-et, the situation is slightly more complicated because 31-et is a prime numbered division. Consequently the antipode of the tonic is the fifth axis -6 -3. In my notation here the -6 means the semi-flat sixth, that is, the "quarter-tone" between 6 and b6. The antipode of a tone is always an axis. Hence we can also say that the antipode of the tonic axis (1 5) is the function -3. Together they form the peculiar neutral triad 1 -3 5. The antipode of an axis is always a tone. In 31-et the antipodal axis -6 -3 is surrounded by a small bounded constellation of functions (the antipodal region) from which we derive the neutral elements - in circle of fifths order -5 -2 -6 -3 -7 +4. These define the alterations that will be applied to the diatonic norms.
Now we are ready to generate our new modes. One example can serve to epitomize the procedure and designation for the whole family. LI = 1 2 3 +4 5 6 7 The shorthand name LI seems to me logically appropriate for this mode, where the +4 mediates between the #4 of L and the 4 of I. Like its diatonic relatives it also forms a major scale. One can ask the question: why choose +4 as the alteration instead of some other function, say -6? The answer is simple but important. We demand that the pattern remains symmetrical. Because I stipulate this property we can generate only a limited number of sets. Negation of symmetry allows the proliferation of many hundreds (no thousands) of sets that are much more difficult to classify and name.
This group is like merely getting your feet wet or just crossing over into the territory. It brings in only one altered element.
LI = 1 2 3 +4 5 6 7 LI and IM form major modes, whereas DA, AP and PO prove minor. Meanwhile, MD is a strange bird neither major nor minor - the first neutral mode. AP exhibits that ubiquitous middle-eastern minor tetrachord 1 -2 b3 4 historically associated with the Greek scientist Ptolemy. Of course, he didn't derive it from 31-et, but rather from the 11-limit just intonation monochord sequence 9:10:11:12. MD embodies the neutral tetrachord 1 2 -3 4. MD deserves further comment regarding symmetry. In the case of 12-et we talk of point symmetry and that's about it. But in 31-et we should also consider axis symmetry about the structurally fundamental tonal axis (1 5). I refer to this property as axis symmetry. In particular, MD exhibits axis symmetry. A critic could argue that the concept of axis symmetry is entirely superfluous - axis symmetry here is entirely equivalent to point symmetry about -3. Although I concede that this is so, I have found the concept of axis symmetry very useful in other contexts, so I retain it here. The reader may notice that only six modes have been given. Where is the seventh? The remaining mode places the tonic on the foreign element of the scale. Thus it reverses the polarity, so to speak, between the antipodes. We get the following climactic pattern in which all six functions are altered. LIMDAPO = 1 -2 -3 +4 -5 -6 -7 Like D, this neutral mode has tonic symmetry. It exhibits somewhat reduced stability, like PO and other modes that embody the -5. For a while I toyed with the idea of naming the whole family the "Limdapo" set of modes - but perhaps this is a bit too weird!
The second structure brings us into the heartland of the territory. Here we find some very useful and strangely beautiful modes with a middle-eastern feel. In this group two foreign elements (one in each tetrachord) alter the diatonic basis.
LIM = 1 2 3 +4 5 6 -7 Only LIM remains major, while DAP and APO preserves the minor. Now we have two neutral modes. Sitting in the middle of the whole group, MDA (like its parent D) has tonic symmetry. Here the antipodal axis -6 -3 has replaced the 6 and b3 of D. Occupying the middle place of the middle group of modes, MDA sits in a special place in the very center of the territory. As in the last group, we must reverse the polarity to generate the remaining two modes, where five elements suffer alteration.
LIMDAP = 1 -2 -3 +4 5 -6 -7 Both form neutral modes. LIMDAP (like MD and IMDA) has axis symmetry. As usual, IMDAPO embodies less stability.
The last group of modes penetrates into the territory as far as we can go. The polarity becomes somewhat blurred. Now we have three altered elements in the scale.
LIMD = 1 2 -3 +4 5 6 -7 Only the less stable DAPO preserves the minor, the rest prove neutral. IMDA (like MD) has axis symmetry. As usual, we can reverse the polarity to get the remaining three modes, bringing four foreign elements into the diatonic structure.
LIMDA = 1 2 -3 +4 5 -6 -7 Now all of these modes are neutral. IMDAP (like D, MDA and LIMDAPO) has tonic symmetry. Having exhausted all of the geometric possibilities on the circle of fifths, this completes the set of twenty-one neutral diatonics.
Early on I indicated that the elegance of the set comes from the restriction to symmetry. However, the astute reader may already have noticed that this condition is necessary but not sufficient. Here is a counter-example: 1 +2 #2 4 5 +6 #6 This septimal mode also has tonic symmetry. Nevertheless, it doesn't qualify for membership because the foreign elements do not come from the antipodal region. -2 sits in the zone but not +2. The pattern also appears kind of "splayed out" or widely dispersed on the circle of fifths. On the other hand, the neutral diatonics all have a certain compactness or compression around the antipode. I must emphasize that my theoretical model comes from the geometrical properties of the circle of fifths. I urge the reader to draw up the circle and observe the region of the antipode. The functions form a tight family (-5 -2 -6 -3 -7 +4) with the natural boundary sentinels at -1 and +1. From there in either direction one aarrives at the septimal zone. I have found the circle of fifths quite illuminating for a variety of theoretical issues. But I will be the first to admit that other approaches are also possible and fruitful. Leo has an alternative, but I won't describe it here. Better to read Leo's paper.
The neutral diatonics prove to be intrinsic to 31-et, but let's expand the horizon a little. They also work equally well within 24-et. This is understandable since both systems describe quarter-tone resolutions of the octave. As a negative example consider 19-et, which is a chromatic (or small) semitone resolution. Here, as in 12-et, the neutral diatonics simply don't exist at all. Like 31-et, 19-et is integrated (which means that it forms a single circle of fifths uniting all the elements). Every integrated system has its own unique antipodal region with its own functional pecularities. Being a prime system, it also embodies both tonal and axis symmetrical structures. 12-et, of course, is also integrated but crude enough to possess only tonal symmetry. However, it also has certain special (reductionist) properties due to the composite nature of the number 12. 31-et and 24-et prove to be close cousins in regard to the acceptance of the neutral diatonics. However, the latter is the poorer relative. 21-et is not integrated. Rather, it forms two independent circles of fifths. How is it then even possible to define an antipodal region for this system? We can still examine symmetry by using the circle in ultra-chromatic order (1 +1 #1/b2 -2 2 etc.) instead of the order of fifths. However, the circle of fifths is much better for examining harmonic properties. 24-et turns out to express only tonic symmetry (like 12-et). 31-et is altogether richer, more complex and intonationally truer than 24-et. Yet, amazingly enough, 24-et expresses the neutral diatonics quite well - just as well as 31-et! One can find a lesson in this situation. We all love our theoretical constructs that prove to be so instructive, even indispensable. However, theory must be grounded in practical experimentation. At the end of the day, practicalities trump theory. Siemen Terpstra, Amsterdam, October 2010
|