
|
On the theory of the art of singing 1)
423
Step is the next subsequent ascent which one rises in natural singing, of which the smaller variety is called minor step, the larger, major step.
Natural singing is that which by an orderly ascent takes place as follows: two major steps, one minor, three major steps, one minor, two major steps, one minor, three major steps, one minor, and so on gradually, in orderly sequence. Since lay people, not knowing the difference between half steps and whole steps, use this progression by natural inclination, it is with good reason called natural singing, because singing two or three half steps, or four or five whole steps in succession not only is difficult to accomplish, but also is unpleasant to hear as well as unnatural.
These seven steps, ascending in an orderly way according to natural singing,
make one round of singing. When one rises seven steps above a given note in an orderly ascent, the last sound is so similar to the first that it seems as if one had made a round and arrived again at where one began, so that, because of that similarity, this is called a round. This is rather similar to what happens in astronomy with the helices described daily by the moon owing to its daily motion, which, though not being properly parallel circles, are so called because of this similarity.
Those seven steps are called as follows: ut, re, mi, fa, sol, la, si, among them the steps from mi to fa and from si to ut are 3) minor steps and all the others major steps.
Two sounds having the same pitch, their relation is called selftone. But differing by a minor step, semitone. Differing by a major step, a whole tone. Differing by one major and one minor step, one-tone-and-half. Differing by two major steps, ditone. And so forth in an orderly way.
1) The selected pages which follow have been taken from the part that is in Stevin's
own handwriting, with the exception of the chapter on the modes.
425
Two sounds having the same pitch, their relation is also called a first (or
prime), but when differing by one step, it is called a second, and when this
step is a minor step, it is properly called a minor second, when it is a major
step, a major second. Likewise, when the sounds differ by two steps, their
relation is called a third, and when one of them is a minor step, it is called
a minor third, but when both are major steps, it is called a major third, and
so on to the seventh, the steps following it being called double-first,
double-second, and so on in a regular way like the simple first, second, and
the following.
Singable sounds receive names of two different kinds, as described in the
foregoing 5th and 6th definitions, each of which has its special use. For when
the ratios of sounds have to be added or subtracted, they are more conveniently
referred to by the names of the tones, since, the tritone added to a ditone,
their sum is a five-tone; the ditone being subtracted from the
three-tone-and-half, the remainder is the one-tone-and-half, in such a way that
sums and remainders receive names in conformity with the numbers to which they
correspond.
We postulate that as one part of a string is to another, so is the coarseness of
the sound of the one to that of the other.
When two persons sing together a double-first, the coarser voice of the lower
has an appearance of doubleness with respect to the sharp voice of the higher,
i.e. as 2 yards are double to 1 yard, so this lower voice in coarseness seems
to be double to the higher. It is true that this doubleness does not present itself
quite so clearly and intelligibly in sound as it does in size, number, weight, time,
motion, and otherwise; yet the stretched string itself induces us to grant this, since
if its parts are in the double ratio as to size, the same sounds ring that we say to
be in the double ratio as to coarseness. For the whole string, when played against
its half, together make us hear the aforesaid double-first. Further, as it has here
been said that, the whole string being to its half in double ratio as to size, its
sound is in double ratio as to coarseness, so also it is to be understood that, the
whole string being to its quarter in a certain ratio as to size, its sound has the
same ratio as to coarseness, and so on for all other cases, parts of the string against
each other as well as parts against the whole string. *)Compositione cantus.
427
All whole tones to be equal and likewise all semitones to be equal.
The meaning is this: that one rises as much from ut to re as from re to mi, and also from fa to sol, from sol to la, and from sa 1) to ut. That likewise one rises as much from mi to fa as from la to sa.
On Ratio in General
Because ratios in the field of sound are not as manifestly known as in other
fields where we meet with them, for the sake of greater clarity we shall first
speak about ratios and equirationality 3) in general; subsequently
about the aspect of ratio in singing by comparison with the familiar ratio in
geometry. And finally of the ratios proper to musical sounds.
1) In the manuscript, erroneously, si. This same error has
been pointed out in note 3 p. 423. Sometimes Stevin writes the scale as
fa sol la sa ut re mi fa. Cf.
figure 1 p. 436.
429
equirationality was not found among the Greeks and their successors. For
(leaving aside many other things, to be discussed elsewhere) from saying that
6, 4, 3 of three sounds make a musical equirationality endless vanities follow
and are concluded.1)
So far we have spoken of ratios in general, but in order to explain now, as intended, ratio in singing by comparison with geometrical ratio, we are to know that, as the geometrical ratio consists in the largeness and smallness of figures, which is measured by length, so ratio in singing consists in the coarseness and sharpness of sounds, which is measured by height or lowness. Thus when two persons sing a double-first, it is said, in view of this difference in lowness of one below the other, that the coarser voice is double below the sharper one. And all the other greater or smaller singing ratios are made of the same stuff as the stuff this doubleness is made of. Again, just as all the ratios of two given rectilinear plane figures or solids cannot be recognized by sight, but obey geometrical rules teaching us how to find them, so all the ratios of two given sounds cannot be judged by hearing, but they are revealed by means of the musical rules governing them, which we now have to discuss.
1) Between two numbers p and q one can have an
arithmetic mean (a), a harmonic
mean (h), and a geometric mean (g). These are defined by p
- a = a - q; 1/p -
1/h = 1/h - 1/q, and p : g =
g : q. Obviously the numbers 6, 4, and 3 quoted by
Stevin show the harmonic mean 4 of the outer terms 6 and 3, 4 being 1/3 more
than 3 and 1/3 less than 6 so that 1/3 - 1/4 = 1/4 - 1/6. Accordingly the note,
given by a length of string 4, is the harmonic mean of the notes given by the
lengths 6 and 3. The latter make an octave. The harmonic mean gives a fifth
against the lower, a fourth against the higher note. Stevin has in mind
geometrical ratio only, and he objects to equating two musical
(singconstighe) ratios to be construed from the three numbers in
question. Obviously he refuses to admit the harmonic ratio to be called a
ratio.
431
Experience shows that a stretched string on some instrument, such as a lute, a
cither, a violin, or the like, produces against its half a sound so similar to it that
it has a semblance of identity, the coarseness ratio of which, by some natural inclination,
we understand to be double, but not with the same evidence as the
doubleness with which we meet in other matters, as has been said before; but this
is confirmed more perceptibly by the bodies producing these sounds, such as the
whole string and its half, which are also in the double ratio. The same also applies
to half the string and its quarter, its eighth, its sixteenth part, etc. in this sequence,
for all these sounds have the aforesaid semblance of identity, with the understandable
form of the fourfold, eightfold, sixteenfold ratio of coarseness. The same is
also obvious in all the ratios besides the above-mentioned sequence. For if we take
a part of the string whose ratio to the whole string is one half of the ratio of the
double part,1) its sound will also have dropped halfway by properly estimated
lowness. But because this known dropping is the measure of the coarseness, as we
have stated above, here the ratio of coarseness is known to us, and the same also
applies to other similar sounds, from which it is concluded that as this part of
the string is to that part of the string, so also is the sound of this to the sound
of that, i.e. the parts of the string produce sounds in the ratio of their
sizes.
1) Stevin means. the square root of 1/2.
433
(as if one should say: the sun may lie, but the clock cannot). They even considered
the sweet and lovely sounds of the minor and the major third and sixth, which
sounded unpleasant in their misdivided melodic line, to be wrong, the more so
because a dislike for inappropriate numbers moved them to do so. But when
Ptolemy afterwards wanted to amend this imperfection, he divided the aforesaid
genus diatonicum in a different way, making a distinction between a major whole
tone in the ratio 9 : 8 and a minor whole tone in the ratio 10 : 9, a difference that
does not exist in nature, for it is obvious that all whole tones are sung equal.
Since these tones of Pythagoras and of Ptolemy displeased Zarlino, he made yet
another division, distributing a certain comma (which remained in Ptolemy's
division) over one tone and another, where it seemed appropriate to him, but
tentatively.1)
1) For this question, see note A, page 460.
435
this being subtracted from 60, there remains 10 for C; adding 10 again to this, he
finds 20 for D; this being subtracted from the 50 of B, there remains 30 for E.
But E was to have 35, so he sees patently that E has not received his due. But
not perceiving that this follows from the fact that the first number for A, i.e. 60,
had not been put right, he considers the last mishap to be the secret of Nature and
looks upon his former supposition as correct. But what will the experienced
arithmetician say to this? Certainly, and with good reason, that such a person is
insufficiently acquainted with the properties of arithmetic, for he knows that the
correct portion for A is 59; when this is subtracted from 110, there remains 51
for B; this from 59, there remains 8 for C; adding another 8, that makes 16 for
D; this subtracted from 51 of B, there remains 35 for E, as required.
But, to come to the point and to describe the proper ratios of natural intervals 1),
I say that the true ratio of the fifth or the three-tone-and-half is 1 to
Above F the double-first f with the fifth c between them 1) Stevin writes: toonen (= tones).
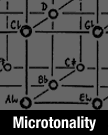
437
Below O the double-first I with the fifth M between them
Figure 1. Plan of a harpsichord's keyboard. Between the keys, semitones supposed minor have been marked c by Stevin (from cleen = small); major semitones by g (from groot = large). Starting the scale with ut on G, Stevin in f has sa, on a white key. This being so, experience shows that H and F make a perfect fifth, and although this is considered a common and certain rule by all those who are skilled in this matter, yet to convince those who should doubt it I thought fit to use the authority of .....1)
Thus from H to F being a perfect fifth, all semitones must needs be equal and
an exact half of a whole tone, which is proved as follows.
1) The same diagram and the method of tuning, in which Stevin uses
the expressions doctaaf (the octave) and de quinte (the fifth)
was shown on a separate leaflet. A foot-note also was on a separate slip of
paper. In the note Stevin supposes that in the tuning experiment one has
started from E-flat (the keys K and P in the diagram). In that case the last
step leads to G-sharp (gis, the keys M and R). Stevin argued that the
people quoted by him proclaim that they find the starting note P to be
identical with the perfect fifth (d-sharp or dis) above M. Hence, he
says, F too is a perfect fifth above H.
439
and from Q to g a minor semitone. Further, O is a fifth above L, by tuning,
therefore from c to O is a major semitone, which is proved as follows: LG is a
minor semitone, Gb two whole tones, bc a minor semitone, making together two
whole tones and two minor semitones. Therefore cO, to make up the fifth LO,
must be a major semitone, and consequently Od is a minor semitone. But
c, O, d
make double-firsts with C, I, D, therefore from C to I is a major semitone and
from I to D a minor semitone. Again, M is a fifth above I, by tuning; therefore
from G to M is a major semitone, for ID is a minor semitone and DE a
whole tone, EF a minor semitone, FG a whole tone, making together two whole
tones and two minor semitones, so that GM, to make up the fifth IM, is a major
semitone, and consequently Ma is a minor semitone. But g, R
make double-firsts with G, M, therefore gR is a major semitone. 1) Mean proportionals, forming a geometric progression.
441
Thus, A,1 to A,1 is the ratio of the selftone or first, but A,1 to C,
*) Inexplicabili irrationali absurdo numero.
443
Now in order to divide the monochord geometrically in such a way that we have the true perfect sounds of natural singing, i.e. in the above mentioned ratios, let AB designate the monochord, whose centre is C. Divide this in D, E, F, G, H, I, K, L, M, N, O in such a way that GB and LB are two mean proportional lines between AB and CB 2), which may be found in practice (for the mathematical method is still unknown) by different methods, but in my opinion most conveniently in the manner of ........
Figure 2. Division of the monochord in equal tones and semitones.
Further in such a way that IB be the mean proportional between AB and CB,
which is found by the .. th Proposition of the .. th Book of Euclid. Likewise EB
between AB and GB. Again DB between AB and EB. Further FB between AB and
IB. Likewise HB between GB and IB; and KB between EB and CB. Again MB
between IB and CB, and NB between LB and CB. Finally OB between NB and
CB.
1) The letters in the first calumn of the following table correspond
to the diagram, fig. I, p. 436. In the second column they refer to the
preceding table on p. 441.
445
So far we have spoken of the geometrical division; in the following we shall
explain the arithmetical division, i.e. by simple numbers, which in practice suffice,
as follows. I divide the line AB into 10 000 equal parts. Now in order to know
how many of these parts pertain to each tone 1), we begin with the
three-tone-and-half, saying: 1 gives
1) Read: interval.
447
One might also perform the aforesaid division as follows. Having found the
ratio of the three-tone-and-half as above, I get that of the tritone by saying:
1 gives
If one now wants to see how far amiss were the erroneous divisions of Pythagoras, BoŽthius, and Zarlino, this is readily possible by putting the largest number of their ratio also 10 000. I take the Pythagorean division, whose table being described up to the three-tone-and-half, runs as follows: 1) In the table a mistake, 8404, has been corrected to 8409. The correct numbers should read:
449
From this it appears that the smallest term, of the three-tone-and-half, is 8 parts too short, for when 6666 is subtracted from 6 674, there remains 8, but the semitone is 54 parts too long. Now one might think: why is this difference so much greater in the semitone than in the three-tone-and-half 1)? I would say that the cause of this is obvious in the example given above in simple language, where we put 110 instead of the six-tone and where five persons, A .... E in due order stood for the three-tone-and-half, the two-tone-and-half, the whole tone, the ditone, and the semitone, where A in the wrong operation received only one too many and B one too few, C two too many, D four too many, but E five too few, so that E had too few five times more than A had too many. And likewise from the same cause the semitone here gets too few, five times more (with respect to the ratio of coarseness) than the three-tone-and-half has too many. From this it is also obvious that the difference between the lesser and the greater semitone is sixteen times 2) greater than the excess in the ratio of the three-tone-and-half, which is the cause of this error becoming so much more perceptible in the semitone than in the other tones.
NoteOne must remember that the names of doubleness 3), triplicity, quadruplicity of firsts, seconds, thirds, etc. do not refer to the coarseness of the sounds, but to the rounds (taking eight 4) successive steps for a round), for just as one may say that two, three, or four turns of a helix are the double, triple, or quadruple of one circumference, not with respect to the unequal lengths of the lines, in which such a ratio does not consist, but with reference to the number of the turns, so these firsts, seconds, etc. are called double, triple, quadruple with respect to the rounds, without minding the coarseness of the sounds, according to which the constituent notes of the triple first are in the fourfold ratio, and those of the quadruple first in the eightfold ratio. With the double-first the matter happens to be different. For a first or selftone is a ratio 1 : 1. Doubling it means adding another ratio 1 : 1 to it; this makes the ratio 1 : 1 again. Therefore the name double-first refers to the rounds of sound only.
1) Stevin explains why the deviation between his scale and the Pythagorean scale
is so much larger for the (minor) semitone (9492 - 9438) than for the fifth (6666 -
6674).
451
Appendix 1)Preface / On the fourth / On la, si, ut / On the twelve modes 2) / In musical composition nature is not followed as it is in Rhetoric / The joint descent and ascent of sixths and thirds is allowed if minor ones alternate with major ones / Why not numerals in the long notes? / Bemollaris cantus is a useless distinction. / It is a common saying that whoso distinguishes well teaches well, but besides it must be known that whoso distinguishes improperly, teaches improperly. Species perfecta and imperfecta is an evil distinction. Having above described the theory of music, I thought it useful to add, in brief words, an explanation of some obscurities and errors rooted in present-day musical practice.
The fourth is considered a discord by present-day composers, so that in singing
with three or more voices it must not be heard against the lowest part; nay,
below two voices it is not suffered at all. But when one asks: why?, they answer:
because it displeases our ears. Which I deny, and I shall also prove the contrary,
first by argument, next - which is more - in practice. 3)
1) Only some of the announced items will be discussed in the
following pages.
453this several times and also several fourths and fifths higher and lower, each asking his partner what it is that he is singing or playing, then we shall find in practice that, judging thereof without certainty, he will frequently contradict himself, often regarding as a fifth what he previously stated to be a fourth, and again conversely judging that to be a fourth which he had previously stated to be a fifth. This being found in practice, what more words do we need? Who is so unreasonable as to disgrace himself by his own words, saying: the fourth displeases me and the fifth pleases me very well? But to set forth the cause of these things somewhat more amply, it must be known that when two such sounds produce together a first or a double-first, the very keenest hearing cannot tell for certain which of the two it is. To speak of this even more clearly by way of example, I suppose that there are two persons, one playing the flute and the other singing, each having sung his part of a given song as one would consider it should be. If this song is thereafter repeated again, but in such a way that the singer goes a double-first higher than before, everyone (for the aforesaid reason, to wit that but for a double-first there is no certainty) thinks it is all right. Nevertheless, at the note where the singer first was a fifth below the flutist, he now inevitably will be a fourth above him, in such a manner that, taking that the first time it was a fifth, one now will hear the fourth as a fifth. Therefore, as to those who still say that the fourth sounds unpleasant in their ears, but the fifth very pleasant, I cannot but conclude that the habit grown during the last century has bred a certain effeminacy in them.
As I intended to speak with Master David about the twelve modes of Zarlino, I copied the notes in my own way, to show him that there were twelve. But though I further meant to prove that there could not be more, I found on the contrary that there were fourteen, the proof of which I am sending you herewith. Therefore, please undeceive me, or be deceived yourself. Zarlino's six modes (which would make twelve with their contraries 2), as you send them to me, are as follows:
Figure 3. The principal notes of the odd numbers of the modes in the Dodekachordon of Glareanus, given to Stevin by a friend who took them from Zarlino.
1) In the manuscript a chapter on the twelve modes, as given by Zarlino, is missing.
We insert, as a substitute, a copy of a letter from Stevin to an unnamed person,
preserved by his son Hendrick. Cf. Note B on p. 461.
455I arrange them in my own way (adding thereto in the seventh place the thirteenth mode), as follows:
Figure 4. The principal notes of the odd numbers of the modes, supplemented by a thirteenth of Stevin's invention. The small figures indicate the numbers of the ecclesiastical modes. Now it is evident that it is by the different places of the semitones that the various kinds of music called different modes are distinguished. Therefore I make seven equal scales, one in each mode, as shown below. By the dotted steps I indicate, for greater clarity, the places which make semitones with their preceding notes.
Figure 5. Places of semitones in the various modes, indicated by the dotted lines. I say that these are all different, which I prove as follows.
457
These modes with their contraries (which contraries I leave aside for the sake
of brevity) make fourteen modes, and there can be no more, for the next, which
would be the fifteenth in the series, would be identical with the first, which I
intended to prove.
The usual distinction that is made between the music that is called Bemollaris and Beduralis is quite useless and is no real distinction, but they are the same thing. For if you give the C sol fa ut key, where B is flattened (Bemollaris), the name of G sol re ut, singing B natural thereto (Beduralis), you have entirely the same music that was written with B-flat. The same is found when the F fa ut key with flattened B is given the name of C sol fa ut with B natural, and likewise when the G sol re ut key with flattened B is given the name of D la sol re with B natural. Or if conversely you give all the latter the name of the former, you will have the same. It is thus no other kind of music than the other, and consequently it is a useless distinction.
What we have said and concluded in a former chapter 6) about the fifth MP is the finding that this same fifth MP is good,*) but it happens in different
1) on d.
459instruments, as experience shows, that at one time it turns out slightly too large, at another time slightly too small, sometimes also good; but because in playing it is used very little, if at all, many masters who tune the instruments leave it to chance, since all the rest that is used is good enough as regards the ear. But to explain the unexplained cause why this fifth on the aforesaid instruments cannot be hit off as right as the natural singing of human voices testifies it should be, the common imperfection should be understood of practical operation in all matters, which cannot be performed as perfectly as mathematical operations. Thus, for instance, when a piece of linen of 50 yards is carefully measured by different people, one will find a strawbreadth, or an inch more than another. But it seldom happens, and then accidentally, that they all arrive altogether and quite alike at the same result. The same also applies in the measurement of surfaces, solids, and other things, such as time, motion, weight, and again in the matter of sound, which is our point in question. For one cannot match two sounds, such as make a fourth, a fifth or a sixth, etc. in such a way that these intervals are quite perfect, unless by chance; nor can they be proved to be so. But to show this practical imperfection clearly, place the fret of a lute in the place where you think its string will make a perfect fifth against another string. After this, shift this fret over the thickness of a hair only, upwards or downwards, and you will find that no appreciable change takes place, although, to be sure, some change does take place. But if you surmise and concede to yourself that you perceive this falseness of the fifth, let the fret be shifted by someone else, in such a way that you do not know whether he shifts it a hair's breadth upwards or downwards, or leaves it in the same place; when he several times thus inquires of you after the goodness of the fifth, in practice you will find your judgment uncertain, often saying that the good fifth is bad and that the bad fifth is good. It is therefore obvious that no human hearing, however keen it may be, is able to fit two tones quite surely in their perfection. From this it follows that many such mistakes, each of which in itself is inappreciable, yet in combination produce an appreciable error. For as in the aforesaid piece of linen measured by various people many small differences in every single yard, added together, finally make an appreciable difference, so here in the case of sounds too. For since this fifth MP is very rarely used, one let these tiny inappreciable errors drift, which together may finally be appreciable, sometimes also inappreciable, as the case may be. Therefore it is not astonishing that superior masters, tuning these instruments carefully, nevertheless in the end find bad notes which ought to be good; this is but natural. And whoso does not understand this, lacks discrimination between practical and mathematical operations, which we had to prove. Simon Stevin, 1585
460
NOTE A, referring to pp. 431/432In his loose reference to Zarlino, tainted with some derision, Stevin does not do justice to the problem Zarlino was trying to solve. Representing the pitches of the common scale by according to the theory of Ptolemy adopted by Zarlino, in the triad c, e, g the note e was the harmonic mean between c and g. The latter making a perfect fifth, c and e make a perfect major third. The triads f, a, c' and g, b, d' were transpositions of the same triad. Thus the numbers assigned to these major chords are 24 : 30 : 36 = 32 : 40 : 48 = 36 : 45 : 54 (= 4 : 5 : 6) respectively. This procedure entails a difference of one comma in the whole tones (d : c) and (e : d), with the ratios 9/8 and 10/9 respectively; likewise in the whole tones (g : f ) and (a : g). It follows that (a : d) is one comma short of a fifth (ratio 3/2). For a transposition of a melody from c : d : e to the initial note g, one therefore needs a note a, one comma sharper than a. This note can be readily produced in singing, but once an organ pipe or a harpsichord string has been tuned to a, it cannot suddenly be brought to a. Zarlino sought for a compromise, by which he might make slight alterations in the pitches that would not disturb the harmonies too much. Suppose we strain the fifth a little bit, by an amount x, and the major third by y, then the pitches of the common scale will become c, (d + 2x), (e + y), (f - x), (g + x), (a - x + y), (b + x + y), c', (d' + 2x). We want the new fifths to be equal, therefore (g + x) - c = (a - x + y) - (d + 2x) (g - c) - (a - d) = -4x + y = comma. Likewise there is equality of the new seconds (d + 2x) - c = (e + y) - (d + 2x), and again (d - c) - (e - d) = y - 4x = comma. Now, if by a kind of equipartition, the fifth being nearly double the third, one chooses for the strains a relation x = 2y, then the solution is x = - 2/7 comma, y = -1/7 comma. This is the solution which Zarlino offered in his Institutioni 1558 (Parte II, cap. 43), and to which Stevin refers. Obviously less damage is done to the fundamental intervals if one puts y = 0, hence x = -1/4 comma. This solution was put forward by Zarlino in his Dimostrationi harmoniche in 1571 (Ragionamento quinto). Full credit for the first description of this solution is given by Barbour to Pietro Aron (Venice, 1523). Stevin does not mention it. For him the problem does not exist, or rather: he puts x = -1/12 comma, this being the difference between his fifth and the Pythagorean fifth of 3/2. Therefore the damage done by his equal temperament to the perfect third of 5/4 amounts to y = 2/3 comma. That solution of the tuning problem was discussed by Zarlino in 1588, in his Sopplementi musicali (Libro quarto, cap. 28). Stevin does not mention this at all.
461
NOTE B, referring to pp. 452/453In this letter, which is not preserved in his own handwriting, Stevin tentatively enumerates two more, i.e. fourteen different modes. In the diagram he indicates by minims (half notes) the principal notes of the odd modes, the so-called authentic modes. These notes are the points of convergence for melodic lines in singing. We believe that Stevin calls tseamval (coincidence) the principal note in the middle which joins the fourth and the fifth that constitute the octave. The fact that, against his usual method, he nowhere gives a definition of this term, shows that his work is not complete. The final and initial note of an authentic, odd mode becomes the principle note in the middle of the following even mode, which is called a plagal mode. It turns out that the first and the eighth mode have the same division of the octave, but the difference lies in the positions of the principal notes. In the third diagram 1), by the added numbers Stevin
Figure 6. The twelve modes of Zarlino, supplemented with numbers 13 and 14 by Stevin. The latter contain in f : b and in b : f' an augmented fourth and a diminished fifth respectively. 1) Fig. 5 on p. 454.
462
indicates the similar octaves. For the even mode in question the minim must be
put one note lower than for the odd mode. Stevin's thirteenth and fourteenth
modes show between the principal notes the discord of a diminished fifth or an
augmented fourth. For this reason they had been rejected by Zarlino.
In the accompanying diagram the editor gives a full exposition of all these
modes (met haer contrariŽn). The editor has also added the title of the chapter
and the line beginning with 4e.8e on p. 454.
463
NOTE C, referring to pp. 456/457
Stevin remarks that one might do without a sign for flat at the beginning of
the stave. By a simple transposition, choosing an appropriate clef, a tune can be
written without such a flat. He says that if you prescribe a b-flat, having c on a
certain line of the stave, then, by changing the clef you can place g on this line.
By the same notes on the same lines, without a flat, the same melody comes out
as before, written with a b-flat.
Figure 7. A simple melody: re mi fa sol la sa - ut la sa sol fa -, where sa has been written as b-flat and as f, respectively.
464
NOTE D, referring to pp. 458/459
This discussion more or less completes the argument in the former chapter on
the true ratios of natural tones, pp. 434-435, where the tuning was described of a
harpsichord. There the tuning started from F and resulted in a tone H (our
A-sharp or B flat) that by ear was to be judged a perfect fifth below F. Graphics created by Ad Davidse.
|
Vande Spiegheling der Singconst
This is the annotated English translation by Adriaan Fokker of
Vande Spiegheling der Singconst ("On the theory of the art of singing")
in Principal Works of Simon Stevin vol. 5, A.D. Fokker (ed.), Amsterdam,
1955-1966, pp. 413-464. The original page numbers have been kept. The even page numbers contained the original Dutch text. |
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||








 (12) 1/2
(12) 1/2 





